1 Introduction

All research involving autism is (arguably) fundamentally tied to the Autism Diagnosis Observation Schedule [ADOS, currently in version 2 (Lord et al., 2000; Gotham et al., 2007; Torres et al., 2013a,b)]. Research spanning disparate fields, from genomics to complex social interactions, relies on this test as the gold standard to classify humans across the lifespan as autistic or on the autism spectrum. Although clinically validated, the ADOS-based diagnosis misses females (D'Mello et al., 2022; Lundstrom et al., 2019; Loomes et al., 2017). Moreover, there are not enough raters to absorb the large number of toddlers, children, and adults who, according to various screening tools, are suspected of being autistic today. The test is long and taxing on both the child and the clinician administering it because it has an average of 27 tasks aimed at engaging the child through social presses and expecting overtures from the child.

The ADOS is a dynamic and flexible test in the sense that the clinician can choose the tasks according to observing the flow of the child’s performance. It also adapts the test on demand, choosing the module that best agrees with the child’s communication level. However, the interaction occurs while the clinician also scores the child’s performance. Though valid to probe social competence, many of the tasks artificially rob the child of a chance to be naturally social, as the interaction is also taxing on the clinician and, at times, awkward and seemingly forced. In this sense, several of the tasks might be biased, interfering with the child’s socio-motor agency and robbing the clinician of the spontaneity characteristic of a natural social exchange. In this sense, we need objective ways to help automate the scoring process and to quantify this potential bias that such a taxing effect may produce on both social agents. New digital means could help us achieve such goals of automation and objectivity, but to be valid, they would need to preserve the clinical criteria, which have been empirically validated across decades. In this sense, clinically informed digital biomarkers that unveil physiological underpinnings of social and communication differences across neurodevelopment could be of use to both clinicians and researchers in the field of autism.

Prior work analyzing thousands of ADOS score records found non-obvious issues with the statistical foundations used to validate this test. While there are theoretical requirements of normality and homogeneous variance in the signal detection theory used to validate the ADOS (Somoza and Mossman, 1991; Hollander and Pena, 2004), as these assumptions are required for independence between bias and sensitivity (Torres et al., 2020b), and to decrease false positives, the empirical data across thousands of records violate these assumptions (Torres et al., 2020b). New methods have then been proposed to help reduce the number of tasks (Bokadia et al., 2020), while also utilizing motor signatures to identify females (Torres et al., 2013b, 2016a, 2017; Bokadia et al., 2020). Several studies related to the sense of agency have addressed interactions in the context of human–computer interfaces (Weiss et al., 2014; Cornelio et al., 2022). However, there are no means to define naturalistic socio-motor agency during physical dyadic interactions and to identify agency in tasks that enhance autonomy in autism (Torres et al., 2013c). Furthermore, no means to implement these tasks using artificial intelligence (AI) and machine learning (ML) methods have been proposed. Such approaches would help us speed up, automate, and scale the assessment process, particularly doing so with respect to currently underdiagnosed females (D'Mello et al., 2022). Moreover, the automated approaches could help us bring the diagnosis to rural and underserved areas, thus helping us diversify our research pool and enrich our therapies.

We reasoned in the present study that the digital ADOS (Bokadia et al., 2020), i.e., the ADOS that is digitally recorded while the child and clinician interact, could leverage the validity of this test as the gold standard for clinical and research use while providing a streamlined version of it that could help us (1) identify objective biometrics of socio-motor agency and (2) automate the process of identifying socially compliant tasks [as defined by the ADOS test (Lord et al., 2000; Gotham et al., 2007; Torres et al., 2013a,b)] using methods from artificial intelligence (AI) and machine learning (ML).

While in current diagnostic criteria, socially compliant tasks are defined as those that are expected based on some set of rules imposed by some sector of society, in our approach, socially compliant tasks are those that provide a sense of agency to the child that is being diagnosed during a social interaction situation. More precisely, we here define social agency as the balance between autonomy and control during a social exchange. We define autonomy as the ability of the child to lead the conversation as much as the clinician does, rather than always following the lead of the clinician. We define control as the ability of the child to effectively predict the consequences of impending social actions and overall behaviors, based on high levels of motor control. The latter means high signal-to-noise ratio and low randomness in the information being exchanged. Both concepts are illustrated in Figure 1 through the evolution of a dyadic interaction. We further develop the concept in the Methods section of the article.

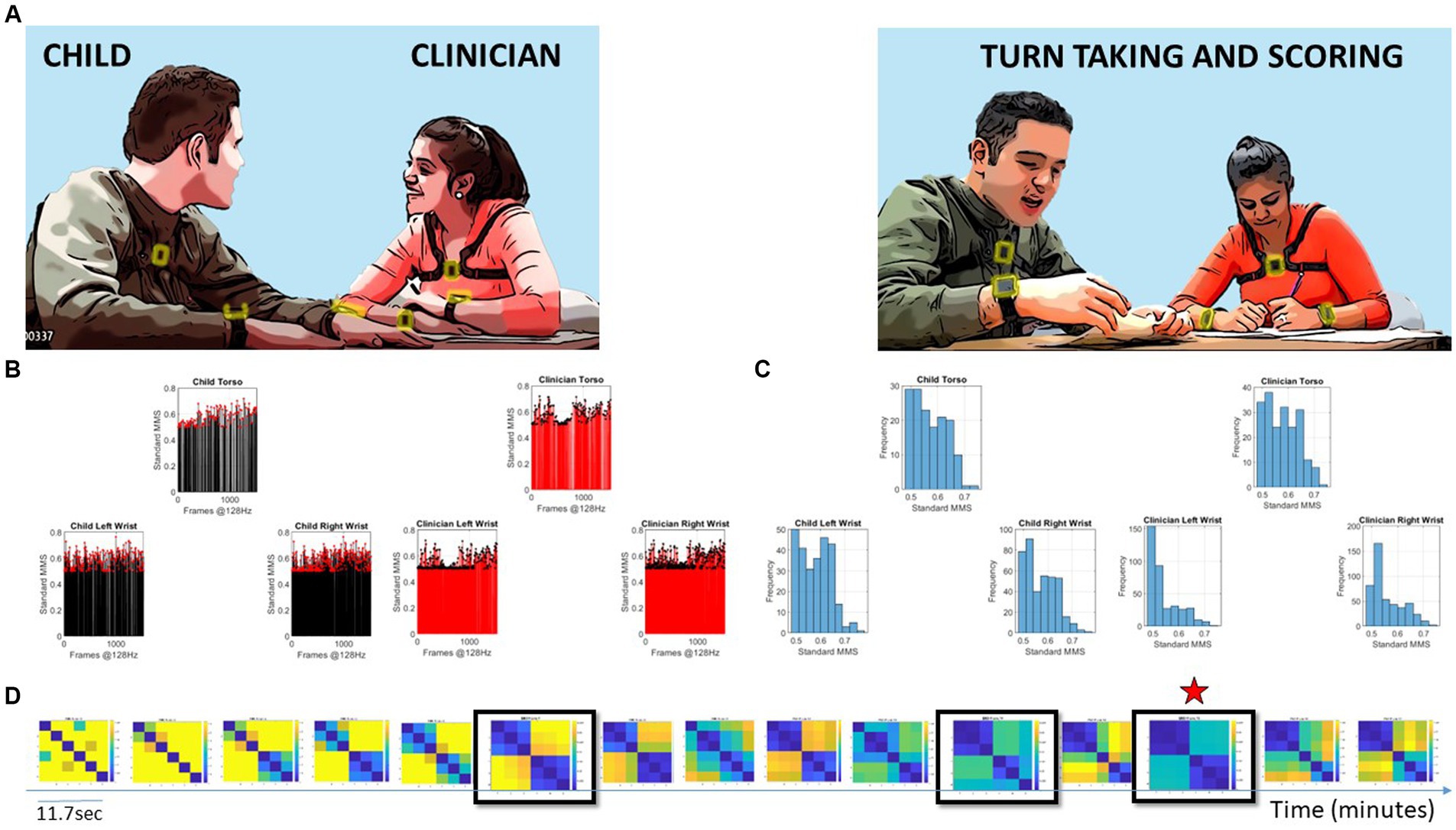
Figure 1. Digitally characterizing socio-motor synergies, rapport, and turn-taking during an ADOS-based social interaction is used here to develop the concept of socio-motor agency through the digital lens. (A) Snapshots of the interaction between a child and clinician wearing six biosensors, three on each body, synchronously registering motion at 128 Hz. Clinician-led interaction and note-taking while rating the interaction. (B) Sample standardized micro-movement spikes (MMS) derived from angular speed capturing approximately 11.7 s of social exchange. (C) Frequency histograms of the MMS peaks (one frame) from each sensor on the child and clinician. (D) Pairwise comparison of the histogram evolution using the Earth Movers’ distance similarity metric. Entries reflect the 6 × 6 matrix (child and clinician, three sensors at the torso, right and left wrist of each) as in (A). Off-diagonal entries are the shared dyadic space, while entries next to the diagonal are the child’s or clinician’s activities in standalone mode. Blue-to-yellow color EMD scale ranks from most to least similar spike coincidence patterns. Star marks the maximal similarity.

Socio-motor agency can be impeded if neurodevelopment undergoes a different maturation path (Torres et al., 2013a, 2023; Brincker and Torres, 2013). If the child, for example, has excessive motor noise and motor randomness in its performance, the predictive ability required for self-motor control will be compromised (Torres et al., 2013a), and with it, the overall control ability will be altered. This alteration will also in turn affect the clinician’s perception of the child’s nuanced micro-motions underlying social behaviors, thus biasing the assessment (Torres et al., 2013a; Bokadia et al., 2020; Brincker and Torres, 2013). Indeed, in autism, there is mounting evidence that motor control is fundamentally different from neurotypical development (Torres and Donnellan, 2015; Torres and Whyatt, 2018; Nayate et al., 2005; Fournier et al., 2010), a fact that has been supported across multiple systems. These include the brainstem (Torres et al., 2023), the cerebellum (Frosch et al., 2022; Allen et al., 2004; Sivalingam and Pandian, 2024; D'Mello and Stoodley, 2015), subcortical structures (Chukoskie et al., 2013), the oculomotor system (Ziv et al., 2024), the reaching and grasping system (Torres et al., 2013a), the gait and balance (Vilensky et al., 1981; Accardo and Barrow, 2015; Wu et al., 2024; Hallett et al., 1993; Bermperidis et al., 2021), among others.

Under such circumstances, socio-motor agency can be impeded, as can be the rating of the child by the clinician. Therefore, we propose that the tasks that manifest excess random noise of the joint dyadic motor patterns (lower control of the dyad) and/or excess lead of the clinician within the dyad (lower autonomy of the child) are inevitably bound to bias the clinician’s scoring toward a deficit model of autism. In contrast, the tasks that manifest high dyadic control and autonomy of the child are bound to boost social agency, according to our biometric definition. These tasks can provide a more appropriate model of readiness potential for social exchange.

The current ADOS is a criterion test that has not characterized neurotypical ranges of observed social behaviors. Furthermore, since motor control physiology is not part of the diagnostic criteria of autism, the test says nothing about neuromotor development. However, the physiology of neuromotor development in formative years provides basic building blocks of social–emotional and communication exchange. Here, we characterize normative data on physiological aspects of motor control. To that end, we study the ADOS exchange patterns in neurotypicals of different ages and provide a new characterization of differences in neuromotor development specific to the social communication criteria that the ADOS offers. The new proposed model can also increase the odds of doing such characterization in a fairer, less biased manner. In this sense, the child is offered a chance to succeed at the exchange. In turn, the clinician can presume competence and identify areas of strength to recommend treatments more appropriately. Such treatments would be grounded on the non-obvious, nuanced aspects of behaviors occurring at a micro-level that escape the naked eye of an observer, in addition to the unambiguous aspects of behavior that such inventories use today. These nuances can be quantifiable with biosensors that read out continuous biorhythmic activities from the nervous system with sub-second resolution. At the same time, they would be informed and guided by clinical criteria.

We here introduce a theoretical framework grounded on empirically derived power (scaling) laws of human ontogenetically orderly maturation on a schedule (Torres et al., 2013a). This framework connects stochastic analysis of human biorhythmic (time series) data with information-theoretical metrics. We define new truly personalized computational indexes of dyadic control, autonomy, and socio-motor agency from biosensors’ digital data using as guidance the digitized ADOS-2. Then, we identify socially compliant tasks, i.e., ADOS-2 tasks with balanced socio-motor agency, thus streamlining the digital ADOS-2. Finally, we propose new ways to help automate and speed up autism screening and detection based on these socially appropriate tasks identified from the motor variability of the interactive dyad, complementing those from the child’s or the clinician’s performance alone.

2 Methods and analyses 2.1 Participants

This study was approved by the Rutgers University Institutional Review Board in compliance with the Helsinki Act. A total of 29 children, including 19 males and 10 females spanning 4–15 years of age, and two adult clinicians participated in the study (see Table 1). Children participated in multiple sessions over the span of 2½ years with one clinician per session and were administered a specific module per session, i.e., a specific subset of ADOS tasks, in accordance with their age, level of development, and spoken language. They were recruited as a random draw of the population, with neurotypicals and autistics signing up for the study through our IRB-approved flyers.

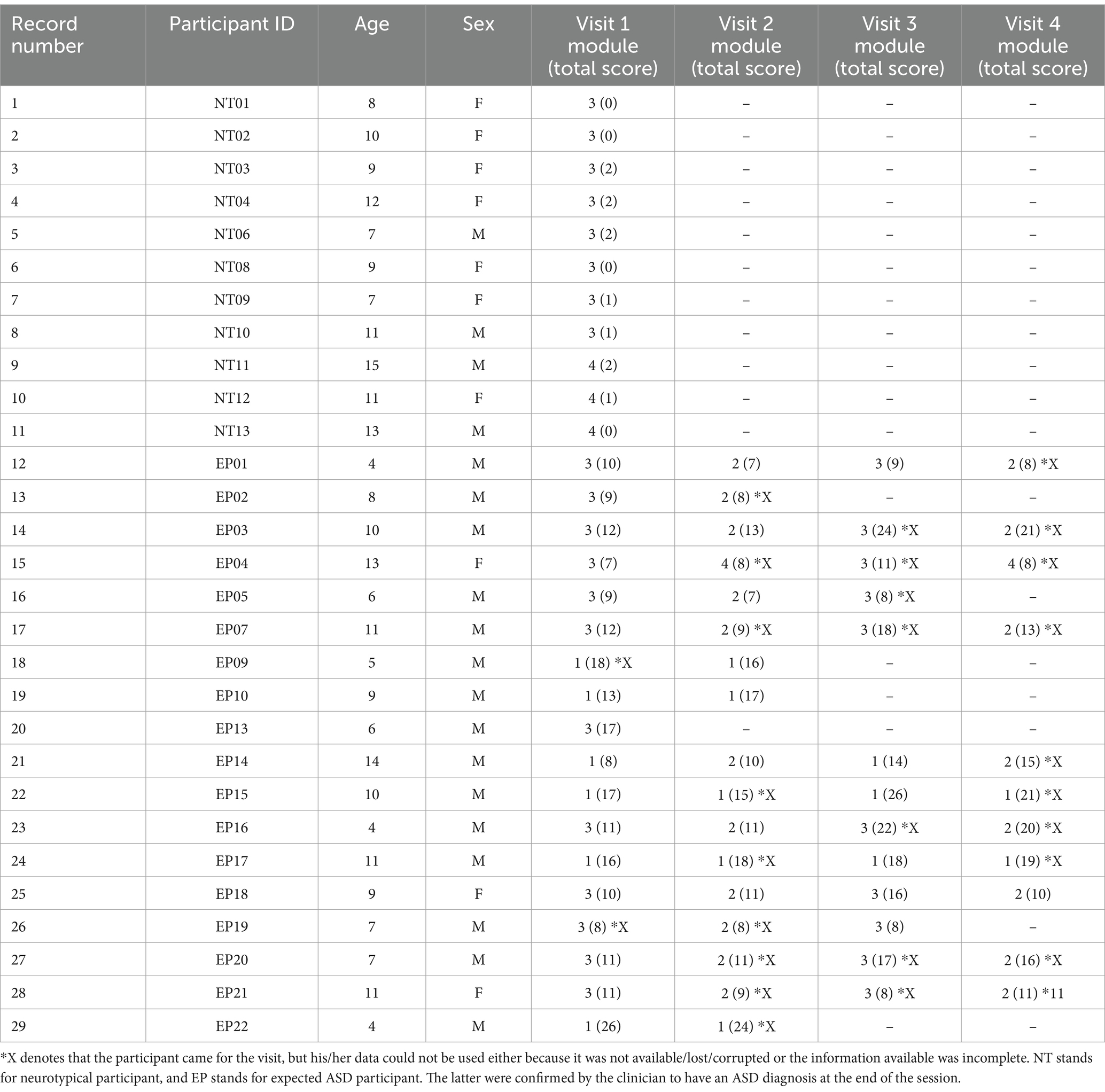
Table 1. Participants’ information.

2.2 Raw data acquisition

Digital data were acquired during each session using light wearable sensors (APDM Opals, Portland, OR, United States). Six sensors were used, two on the left and right wrist and one on the torso, both on the child and clinician. The sensors continuously and synchronously recorded triaxial accelerometry and gyroscopic data at a sampling frequency of 128 Hz. The recording environment followed the standardized ADOS requirements using similar table and sitting arrangements for the clinician–child dyad. The two clinicians were unaware of the goals of the study.

2.3 Data type: the micro-movement spikes derivation

The micro-movement spikes (MMS) are a data type that we invented (Torres et al., 2013a) to create a standardized time series representing the moment-to-moment fluctuations in signal amplitude and timing relative to an empirically estimated mean while scaling out allometric effects. Allometric effects are due to anatomical disparities across participants (Lleonart et al., 2000). Anatomical disparities influence the kinematic parameters, and as such, confound the results from the analyses (Torres et al., 2018). The MMS can be obtained from any biorhythmic activity registered with biosensors or cameras, consisting of peaks and valleys changing over time. For example, they can be time series of triaxial acceleration from accelerometers (inertial measurement units) or from triaxial angular velocity obtained with gyroscopes. In the present study, we focus on the latter. Scalar values of angular speed from orientation data that the gyroscopes recorded were acquired using the Euclidean norm (Equation 1) of the coordinate components of motion as measured by the sensors:

From here onward, all analyses refer to the scalar value V, the angular speed in deg/s.

To scale out allometric effects, we normalize motion data fluctuations (peaks and valleys) (using Equation 2) as relative deviations from the empirically estimated mean activity of the series. To that end, we use maximum likelihood estimation (MLE), and upon testing various families, we obtained the shape and scale parameters of the continuous Gamma family of probability distribution functions. From these empirically estimated parameters, we obtain the moments and use the Gamma mean to shift and center our data around the estimated mean. Then, we obtain the local absolute deviations from the empirically estimated Gamma mean. We scale the mean-relative deviations to the [0,1] interval according to the local minima average:

NormPeak=PeakPeak+Avgmintomin    (2)

Here, 0 values represent 0-deviations from the empirical mean of the session. They are “quiet moments” relative to the person’s mean. They are the baseline value of the individual and provide a personalized signature that changes over time or with the context. For this reason, we focus on the relative deviations from this individual’s baseline. Values away from 0 value represent activity above the person’s baseline mean within the given situation. The normalized peak series of MMS (Torres et al., 2013a; Wu et al., 2018) conserve the temporal structure of the original speed/acceleration time series but are normalized (Torres et al., 2018). This normalization provides standard ranges for each person and permits comparison of motor biorhythmic patterns across individuals, independent of their age differences and corresponding disparate anatomical lengths. Supplementary Figure S1 provides the pipeline to compute the MMS.

2.4 The gamma process of the MMS

The normalized angular speed MMS is best fit (in the MLE sense) by the continuous Gamma family of probability distributions (Torres et al., 2013a; Torres, 2011a). Furthermore, the parameters of the Gamma distribution, shape k, and scale θ have been found across multiple studies from our laboratory, including the present one (see Results), to follow a scaling power law of the form described in Equation 3:

k≅aθb→logk=a+blogθ+ε    (3)

where ε is a small error term and b < 0. This power law for the standardized MMS time series reveals a maturation process of the motor code for voluntary (Torres et al., 2013a, 2016a) and involuntary (Torres et al., 2020a) motions. This law is very important because it provides us with a quantitative framework to interpret fluctuations in biorhythmic data that range from random to predictive. Furthermore, they offer a form of ground truth to quantify deviations across the human lifespan. Because of this tight linear relation of the log scale and log shape, knowing one helps us infer the other with high certainty.

Importantly, the continuous Gamma family of probability distributions has the first (mean) and second (variance) moments expressed in terms of the shape and scale described by Equation 4).

μ=kθ,σ2=kθ2    (4)

Then, using Equation 4, the noise-to-signal ratio (NSR) of the MMS reduces to the Gamma scale parameter as in Equation 5:

NSR=σ2μ=kθ2kθ=θ    (5)

The Gamma scale parameter in Equation 5 fully characterizes the noise of the motor patterns of the interactive dyad (or of the participant), i.e., in relation to the level of fluctuations of angular speed during the ADOS activities.

Empirical estimation of these parameters in thousands of participants over more than a decade of work involving humans along the lifespan, and across disorders of the nervous system, has revealed an interpretation for the Gamma log–log parameter plane. Distributions that fall along high NSR regimes are also close to the memoryless random regime of the special exponential distribution case (when k = 1). Points in mid-NSR correspond to heavy-tailed Gamma distributions. Then, low NSR (or high signal = 1/NSR) are congruent with symmetric shapes (Gaussian-like) distributions. High-signal Gaussian regimens are highly predictable in contrast to high-noise memoryless random exponential regimes. As such, this parameter plane is empirically interpretable. Supplementary Figure S2 shows the Gamma process interpretable parameter spaces.

2.5 Quantifying motor control from the perspective of an agent

Noise-to-signal ratio measures the degree of motion variability away from mean activity. Low regimes of NSR characterize steady and smooth motion, akin to goal-oriented behavior, as experienced from the perspective of the agent/child. On the other hand, high NSR regimes indicate unpredictable and random motion. This is so because of the scaling power law, since knowing one parameter infers the other. At high NSR, the tendency of the distributions is toward the memoryless exponential, representing randomness. In that sense, the high NSR is a proxy for low motor control. In contrast, high signal (or low NSR) infers the existence of predictable motor patterns toward the Gaussian regimes of the Gamma process. Because the NSR is calculated on the standardized MMS, motor noise does not depend on the anatomy of the individual as it is scaled by the mean amplitude of motion. This enables us to plot all participants across different ages on the same parameter space.

2.6 An information theoretic approach to the analyses of the MMS

The presence of MMS peaks indicates an outburst of activity away from the individual’s baseline. This is informative of motor activity within a given context, which in this case was maintained similarly across participants, as per ADOS requirements. When we also consider the temporal distribution of MMS peaks, a train of such spikes can be viewed as a representation of information regarding human motion variability through time. Considering multiple sensors sampling in synchrony, the MMS spikes carry spatiotemporal information about the bursts of distributed bodily activity in the person’s motor system.

Following our argument, we redefine socio-motor agency as the balance between motor control and motor autonomy, using these physiologically grounded biosensor data. Signal-to-noise ratio characterizes the ability of an agent to (internally) control their own motor behavior. Entropy rate characterizes the ability of the agent to motorically act autonomously (while minimizing external control by another agent) in a social interaction.

Finally, using transfer entropy, we can quantify the amount of causal influence from the clinician to the child and vice versa, without the need to use any model or make any other assumptions. Figure 2 depicts these proposed concepts in schematic form.

Figure 2. Digitization of the Autism Diagnostic Observation Schedule (ADOS): Angular speed samples (128 Hz) from wearable sensors on the wrists and torso of the child and clinician are normalized and binarized to obtain discrete sequences of 0’s and 1’s. Entropy rate estimates measure the unpredictability of the underlying binary processes to characterize the agents’ autonomy in the dyadic social interaction. The analysis is performed on data from time windows of ~7.8 s, which proved optimal to attain tight 95% confidence intervals.

The main concepts and formulas that we introduced to characterize control and autonomy can be summarized in Table 2, and we refer the reader to the Supplementary material where we further expand on these concepts and define the clinically relevant parameters as well.

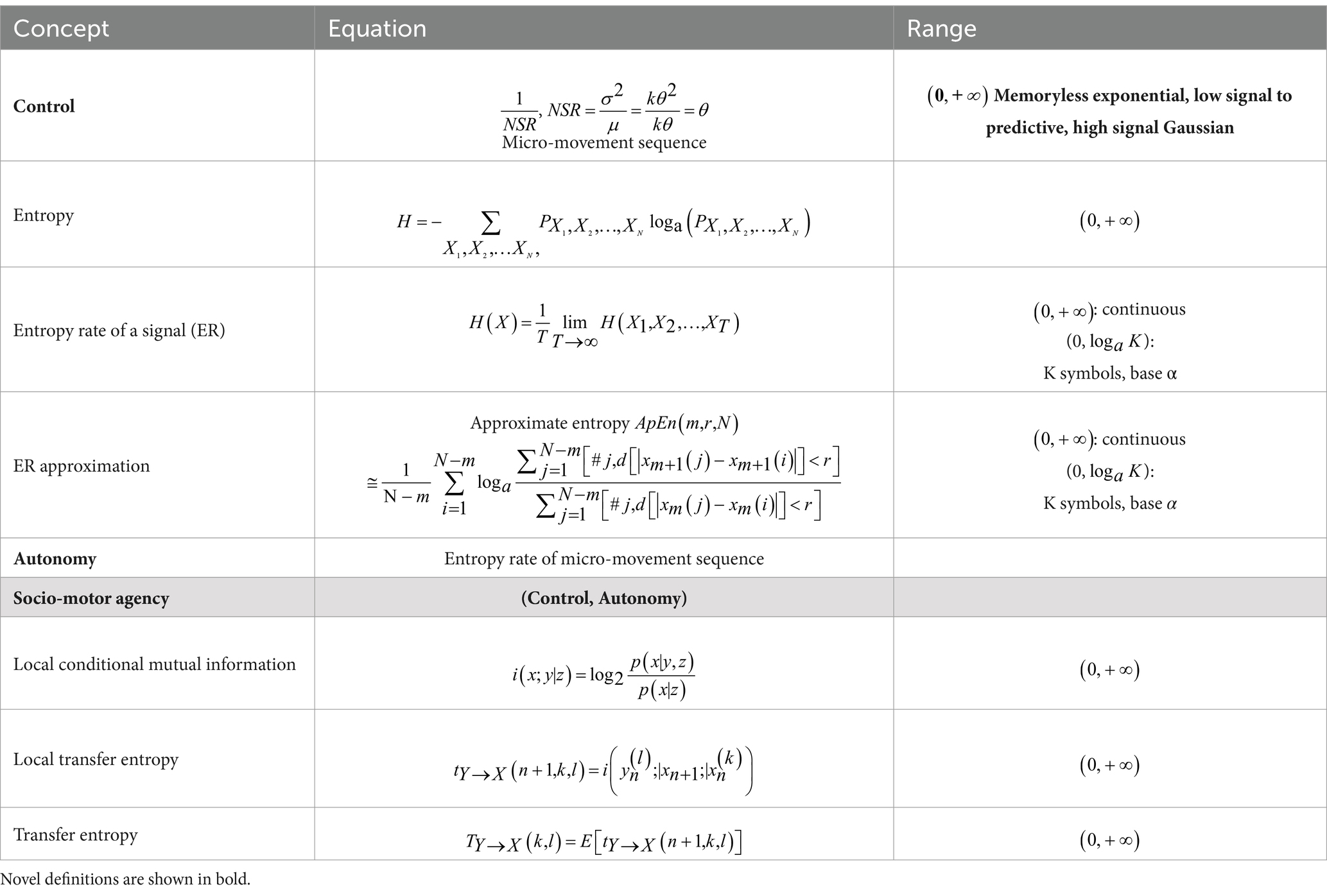
Table 2. Glossary of main concepts, formulas, and their respective domains.

For more in-depth details on information theoretical concepts, formulations, and models that were used in this study, please consult Chapter 1 of the Supplementary material.

3 Results and discussion 3.1 Age-dependent dyadic motor control separates neurotypical (NT) from children with autism spectrum disorders (ASD)

The empirically estimated Gamma parameters localized each child–clinician’s dyadic interaction for each task on the Gamma parameter plane with 95% confidence intervals (Figure 3A). These points represent the empirical probability density function (PDF) of their joint dyadic interaction. When we plot the full scatter estimated from each task in the ADOS for all children, a tight linear relation emerged whereby the log–log plot follows a scaling power law of the form k≅aθb . [see Methods for a more in-depth analysis of the power law and the micro-movement spikes (MMS) time series transformation].

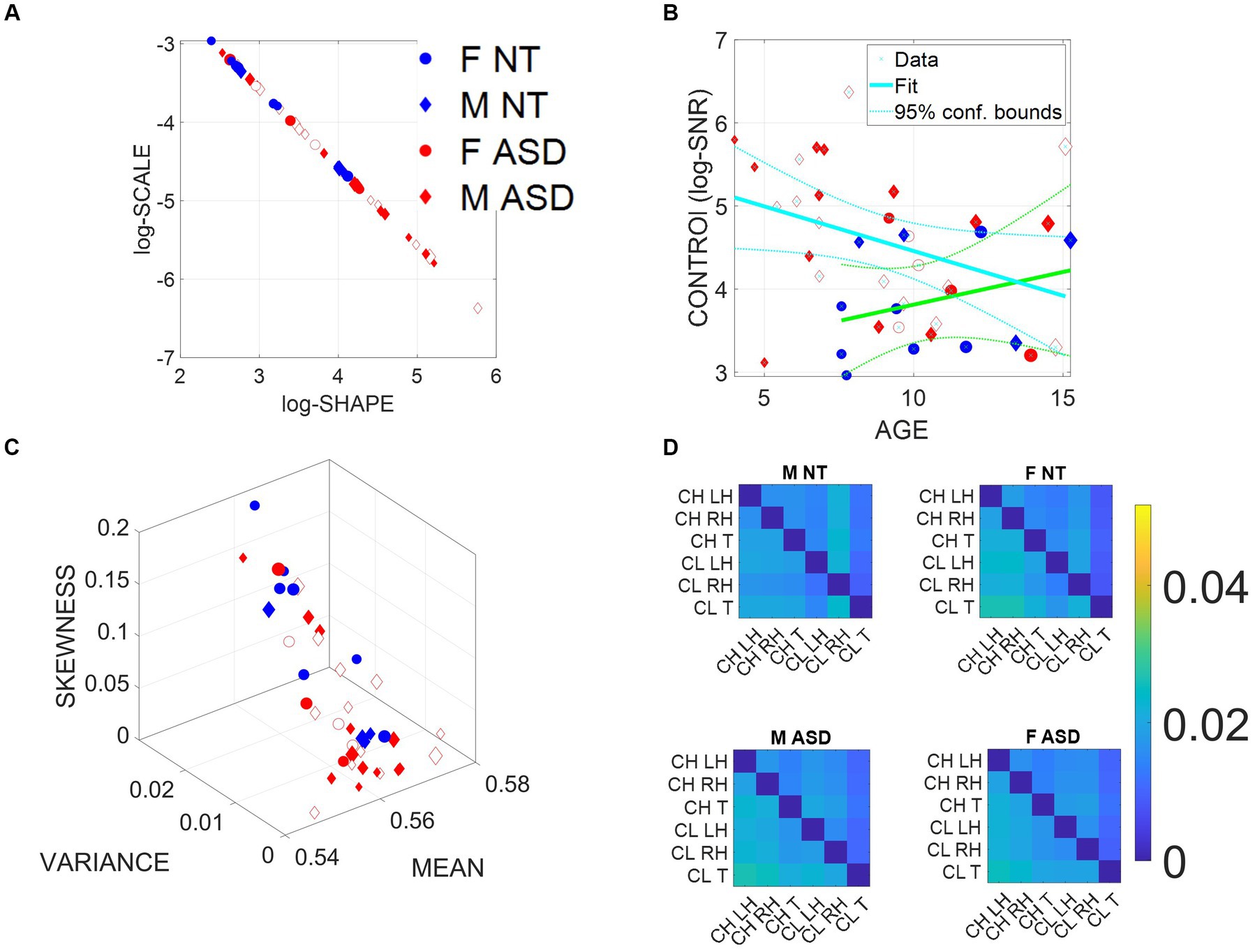
Figure 3. Summary of stochastic characterization of micro-movement spikes, MMS, derived from ADOS-driven dyadic interactions, using angular speed registered from the right (dominant) wrist. Activity encompasses the entire ADOS session. Filled markers represent first visits to the clinician; unfilled markers are subsequent visits. (A) Empirically estimated Gamma Shape and Scale (NSR) for each participant using Modules 1, 3, and 4 of the ADOS test as a backdrop behavioral assay. The size of the marker is proportional to the age of the participants. Empirical Gamma plane of individual children and clinicians separating young from older children and adults in an interpretable map of human neuromotor maturation. (B) Child’s negative Gamma scale parameter (−log NSR = log SNR) denotes control as a function of age. Observe (cyan line) that after school age, there is a decreasing trend of SNR with age for ASD in contrast to the opposite trend for NT (green line). (C) Parameter space spanned by the empirically estimated Gamma mean (x-axis), standard deviation (y-axis), and skewness (z-axis) derived in (A). Marker size is proportional to age. (D) Quantification of transfer entropy for social dyads involving clinician and child obtained for males and females in the NT and the ASD groups, using six sensors, three on the clinician and three on the child, outputting time series of angular speed motion on the left and right wrists and the trunk of each social agent in the dyad. Off-diagonal entries represent joint dyadic activities.

This relationship, first described as a maturation law in humans’ voluntary decision-making, mediated by pointing motions (Torres et al., 2013a), is reproduced here for gyroscopic data reflecting joint dyadic angular speed, such that as the Gamma scale value decreases, the Gamma shape value increases. Because knowing one, we can predict the other with high certainty, we can then reduce these two parameters of interest to one parameter summarizing these motor signatures of the interacting dyad. We can also do so for each individual signature, i.e., those of the child and those of the clinician in standalone mode.

Importantly, the continuous Gamma family of probability distributions has the first (mean) and second (variance) moments expressed in terms of the shape and scale as in Equations 4, 5:

The Gamma scale parameter in Equation 5 fully characterizes the noise of the biorhythmic motor patterns of the interactive dyad, i.e., in relation to their joint level of fluctuations of angular speed during the ADOS activities.

Prior research from our lab concerning autonomic (Elsayed and Torres, 2023), involuntary (Torres and Denisova, 2016), automatic (Bermperidis et al., 2021), and voluntary movements (Torres et al., 2013a; Wu et al., 2018) and research from other labs involving the oculomotor systems (Ziv et al., 2024) has consistently shown across levels of functionalities and systems that high noise levels and randomness correlate with levels of autism severity. Specifically, high-signal Gaussian regimens are highly predictable in contrast to high-noise memoryless random Exponential regimes.

The Gamma parameter plane, which is empirically interpretable, provides criteria for the locations of the Gamma distribution shape and scale parameters. They characterize the signatures of individual participants and change in an ontogenetically orderly manner whereby a decrease in the NSR is accompanied by a decrease in randomness (away from the memoryless exponential distribution at shape = 1), and this decrease, in turn, corresponds to human neurodevelopmental maturation.

The stunting of maturation of the human nervous system has then been well characterized by high NSR and random fluctuations previously found across different ages in ASD (Torres et al., 2013a). In this sense, we equate high SNR = 1/NSR with an index of controllability. As per the scaling power law, high SNR of the MMS is equated with high predictability of the person’s self-referenced, self-generated motor code. Our lab has proposed that this motor code represents a proxy of kinesthetic reafference, i.e., the continuous stream of re-entrant motor activity from the periphery, serving as an index reflecting the quality of the motor feedback to the central controller of the nervous system (Torres et al., 2013a; Brincker and Torres, 2013).

Then, as this motor code is shared with another agent during social dyadic interactions, the distributions of the joint dyadic interactions of the participant and the clinician for the 26 participants (11 neurotypically developing NT and 15 ASD) can be appreciated in Figure 3A following a power law. These distributions are derived from the MMS that fluctuations in angular speed produced in the dominant hand (see Methods; Figure 2).

Furthermore, Figure 3B shows that the log (SNR) = −log(NSR) (denoted as the index of control) of the interacting socio-motor dyad has an age-dependent pattern. In NT children, as the age increases, control tends to slightly increase, i.e., a slight positive trend is reflected in the slope of the line fitting the (blue NT scatter), NT: intercept = 3.0271 p = 0.0067, slope = 0.0786, p = 0.362. In contrast, as ASD children age, control tends to decrease, i.e., a strong negative trend is quantified in the slope of the line best fitting the red ASD scatter: intercept = 5.5317, p = 5.65 × 10−12, slope = −0.1074, p = 0.0463.

In Figure 3C, we compare the two groups by localizing each participant on the Gamma moments space spanned by the empirical mean, variance, and skewness, whereby each point represents the empirically estimated moments of the Gamma PDF of joint dyadic activity for each child–clinician pair. This result demonstrates a tendency of the joint dyad moments to separate NTs from ASD participants, as they interact with an adult clinician, expressing marked differences between males and females.

To better appreciate the sex differences, we obtain pairwise the transfer entropy (TE) from child to clinician and from clinician to child. See Methods for an extended definition, but recall that TE is the reduction in uncertainty of predicting the future of X when we consider the process Y. In Figure 3D, we can see for each matrix the pattern that emerges when considering the time series data from each of the six sensors attached to the child’s and clinician’s two hands and trunk. The cross terms in the off-diagonal entries of the matrix (top right-hand entries 1,4 to 1,6; 2,4 to 2,6; 3,4 to 3,6; and bottom left-hand entries 4,1 to 6,1; 4,2 to 6,2; and 4,3 to 6,3) represent the dyadic cases of child to clinician and clinician to child, respectively. In the shared entries of the matrix, we see that in ASD, males show a decrease in TE values while females show an increase. In the context of the ADOS, females evoke a reduction in the clinician’s uncertainty predicting the impending females’ motions, i.e., perhaps an inherent bias that partly accounts for the disparate ratio of approximately 4–5 males per female diagnosed with ASD (D'Mello et al., 2022). We will further explore these differences to try and understand the interplay between the NSR as an index of motor controllability (predictability) and the overall sense of socio-motor agency in each of the ADOS tasks, for males and females.

In the diagonal sub-matrices (top left-hand entries 1,1 to 1,3; 2,1 to 2,3; 3,1 to 3,3; and bottom right-hand entries 4,4 to 4,6; 5,4 to 5,6; and 6,4 to 6,6), we represent the patterns within the individual’s body parts. There we appreciate higher values of TE from child to child in both ASD males and females, with ASD females having higher TE than ASD males. As with the shared dyadic activity, here in the individual patterns, the highest differences for clinician to clinician can be appreciated in the ASD females.

3.2 Quantifying dyadic social agency reveals differences between NT and ASD

High levels of NSR in the MMS fluctuations from the angular speed coincide with memoryless random regimes of motor patterns—well characterized by the exponential distribution previously found in autistic individuals (Torres et al., 2013a; Torres, 2011b). It has been proposed that under such random and noisy motor code, it is difficult to have high-quality motor feedback contributing to a predictive code. Such predictive code would be necessary to compensate for sensory-motor and inertial time delays inherent in the nervous system (Torres et al., 2013a; Brincker and Torres, 2013, 2018; Torres, 2011b; Mohamed Thangal and Donelan, 2020).

In a dynamic dyadic social interaction such as that taking place during the ADOS, it is then difficult to exert control over the interaction because presses by the clinician and overtures by the child are not occurring at the expected timely rates. This temporal mismatch in autism alone can bias the rating by the clinician in ways that differ between NT and ASD but also may differ between males and females. Here, we equate high NSR with low predictive control and posit that the type of socio-motor agency required in a naturalistic social interaction will be impacted by poor controllability levels on one side of the dyad. We then question whether dyadic-based control (i.e., shared by the child and clinician) is differentially impacted in ASD participants.

Another aspect of dyadic social agency is motor autonomy. As mentioned earlier, motor autonomy is defined here as the ability of the child to lead the conversation as much as the clinician does, rather than always follow the lead of the clinician. An obvious way to quantify the degree to which the clinician is leading the exchange would be by using some form of causal analysis between data recorded on the clinician and data recorded on the child. As our main approach, however, we choose to quantify autonomy in ways that depend on data recorded from wearable sensors on a single agent which as we will show, is intuitive and can be applied in a clinical setting, to help digitize the ADOS.

We introduce (behavioral) spike trains from the MMS derived from the time series of angular speed. We use entropy metrics to examine the degree to which the spikes behave randomly or deterministically (i.e., containing periodic, systematic patterns). To that end, we use entropy rate, a metric well suited to interrogate the stochastic regimes of spike trains (Delgado-Bonal and Marshak, 2019; Lizier, 2014).

From the MMS derived from time series of angular speed, recorded either from the left or the right hand of the child, we derive binary sequences whereby a sequence of 1’s corresponds to bouts of activity and 0’s to “quiet” sampling periods, when no significant change above the person’s average activity occurs in the angular speed profile. Another way to view these binary sequences is as the manifestation of an underlying “alphabet” that characterizes the predictability of the motor code. Zeros and ones will appear with some probability, which we expect to change at some time scale due to the non-stationary nature of human motion. But if we restrict ourselves to small time windows (~7.8 s, determined as optimal for empirically estimated confidence intervals, upon sampling different sizes), this time window is small enough that the process can be viewed as stationary yet large enough to contain a satisfactory number of samples, lending statistical power to our empirical estimation per window. As such, 7.8 s is our unit of time for the spike trains that we derived. Using this MMS per unit of time as our data type, we can then measure the degree of randomness of the child’s motions by estimating the entropy rate (see Methods). Furthermore, we then compare it to transfer entropy (TE) obtained from the child and clinician, a causal metric that can inform us of who leads the interaction for any given task.

We argue that a suitable scale of motor autonomy is one in which, at one extreme, a high degree of randomness is a measure of a system at its highest degree of motor autonomy. This is the type of state where the system is uncontrollably “hidden” from the controller. There is no opportunity to control the person. At the other end, the lowest degree of randomness leads to a systematic, deterministic pattern, highly controllable. While in the former, the child’s system with excessive autonomy prevents social exchange with the clinician in that the clinician cannot control the child, in the latter, the clinician can absolutely control the child. Either extreme is detrimental to the development of rapport or turn-taking in a social exchange. A happy medium is one in which, while the child preserves a degree of autonomy that enables a balanced social exchange, the clinician also partakes in a give-and-take interaction rather than leading the child most of the time.

We test our new hypothesis that motor autonomy relates to measures of entropy by comparing TE (a measure of causality) from the child to the clinician with entropy rate, a measure spanning a scale from totally random to totally deterministic states of the spike-based code. We show in Figure 4A an age-dependent trend spanning two scatters. In older neurotypical children, the scatter aligns such that as the child’s entropy rate (denoting our scale of motor autonomy) increases, so does the TE, denoting a causal lead of the child over the clinician. In contrast, a second scatter emerges for younger children, whereby the trend is less visible, indicating that these children’s index of motor autonomy is not as evident during the exchange and the causal lead (TE), denoting the child’s lead over the clinician’s lead, is less evident. We can appreciate the shift in this metric of motor autonomy in Figure 4B, where the histogram of the ASD children is shifted to the left, indicating lower density values than NT children.

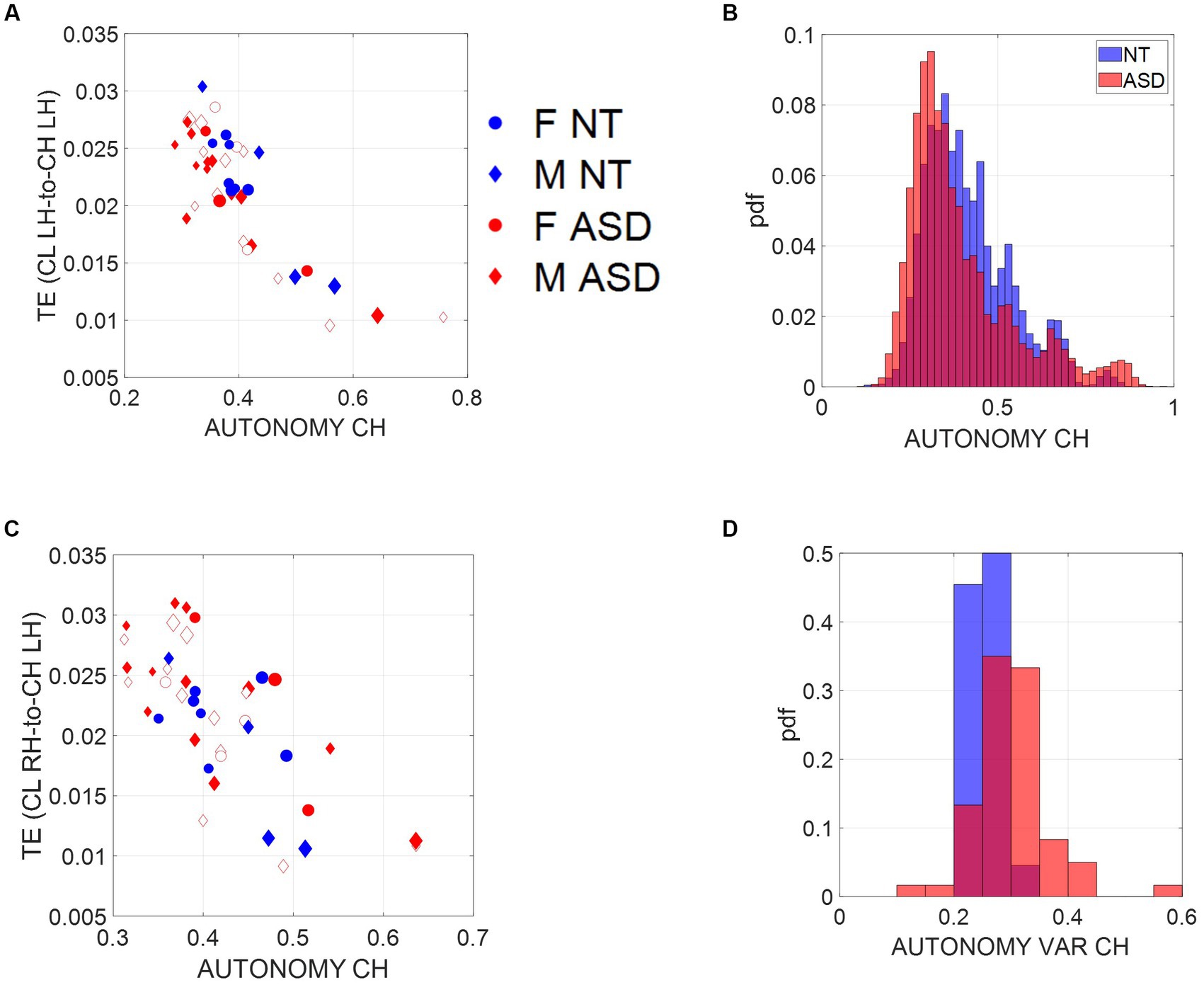
Figure 4. Scales of socio-motor agency according to index of autonomy. (A) Average transfer entropies between child and clinician taken over windows of ~7.8-s duration, per participant (filled-in markers are first visit to the clinician, unfilled markers are subsequent visits) versus autonomy (Ap Ent) reveal higher autonomy index in NT, a trend that is also quantified in (B). As the child’s autonomy decreases, the CL to CH TE (measured in left hand) increases. Adding the CL past activity does not contribute more information about the CH state than looking at the CH past activity alone. (C) This is also the case for the right hand. (D) Autonomy variability (variance over the mean) throughout a session is higher for the ASD group, including both the child and the clinician involved.

Since the left hand is not the dominant hand in these children, we plotted the histograms pertaining to the left hand as well (Figure 4A, left hand and Figure 4C, right hand) to see if these effects consistently emerged. We see in Figure 4B that across multiple time windows, the pdf for the neurotypical group is shifted to the right, meaning that on average, NTs have higher values of autonomy than ASDs. Since autonomy also varies throughout sessions, plotting the autonomy variability (variance of this index over the mean of this index) for different participants in Figure 4D shows that for the ASD group, child and clinician variability is higher than most NTs. This variability index tends to separate ASD from NT participants, particularly for later visits (as the child aged, over 2 years and a half that the study spanned).

3.3 Age-dependent motor autonomy across children versus clinician’s motor autonomy robustness

As we saw earlier, the SNR (1/NSR) of the control index has a trend with age that differs between the two groups. NT children show increasing control with age, whereas ASD children show a decreasing trend. Similarly, here we ask if the index of motor autonomy also changes with age. To that end, we examine this index as a function of age across the children. We also examine it for the mature adult clinician across the children’s ages.

We find that the child’s index of autonomy for both NT and ASD increases with age in all cases (Figure 5A). This result reveals that the ability of the ASD child to actively participate in a dyadic interaction is a human socio-motor developmental trait that improves with age. In contrast, Figure 5B shows that the clinician’s autonomy is independent of the child’s age. In this case, the adult clinician shows no discernable trend.

Figure 5. Non-equivalence of the index of motor autonomy and the index of motor control. Plots reflect the average child and clinician index of motor autonomy for left-hand motions versus age as well as right-versus left-hand index of motor autonomy, index of motor autonomy variability, and index of motor control. (A) Child index of motor autonomy is positively and linearly correlated with age. (B) There is no trend between the clinician index of motor autonomy across children’s ages. (C) Equivalence of index of motor autonomy derived from the left hand versus the right-hand motions. (D) Index of motor autonomy variability also correlates between the two hands and separates NT (blue) versus ASD (red). (E) No definite relationship between index of motor control and index of motor autonomy is observed; however, for small values of motor control index there seems to be a positive trend with autonomy, which then becomes negative for high values of motor control. (F) Left-hand motions have higher variability in the index of motor control than do right-hand motions. Notice the independence from (C), representing autonomy.

3.4 Indexes of autonomy and control are not equivalent

The child’s index of motor autonomy and the variability of this index extracted from the sensors in both hands are linearly correlated (Figure 5C). In the case of the index of motor control, however, there is higher variability of the mean motor autonomy index across subjects when we use data from the left-hand sensor (as shown in Figure 5D, where separation of the NT from ASD is evident). For this reason, we focused our analysis on the non-dominant, left-hand motions. Furthermore, the index of motor autonomy derived from the left-hand motions as well as its index of motor control are positively correlated for small values of motor control index but negatively correlated for higher values. This is shown in Figure 5E. In other words, autonomy and control are not equivalent metrics. This can be further appreciated in Figure 5D (motor autonomy variability) versus Figure 5F (index of motor control).

3.5 Male versus females respond differently to ADOS tasks—the case of ASD females

In addition to the quantification of indexes of motor control and motor autonomy as components of socio-motor agency, we rendered it important to consider the heterogeneity of tasks in the ADOS’ modules 1, 3, and 4 used here across children with different levels of spoken language. We grouped tasks into three main categories: Socio-Motor, requiring high motoric components (frequent movements and gestures); Abstract, tasks more “mental” in nature, requiring abstraction, theory of mind, and other cognitive components; Emotional, tasks that elicit feelings and emotional reactions, strongly visibly impacting the child’s emotional states.

We calculated the average indexes of motor autonomy and motor control across all participants, derived from samples corresponding to the different ADOS tasks. Then, we assessed potential differences between ASD and NT participants, focusing on the comparison of males versus females. We found that ASD males respond with lower index of motor autonomy than do NT males. In contrast, ASD females versus NT females, manifest very modest differences, inclusive of three tasks with no significant differences [Social Difficulties and Annoyance, Loneliness (both Emotional type tasks), and Construction Task (Socio-motor type task)].

It is therefore clear that ADOS tasks inherently bear a lack of differentiation between NT and ASD females, unlike their male counterparts, for which the differences are large. This can be appreciated in Figures 6A,B (females) and Figures 6C,D (males), where we color code the task type and code it numerically according to the name of the task (the Methods section describes the ADOS tasks included from each module). Modest differences were observed between females.

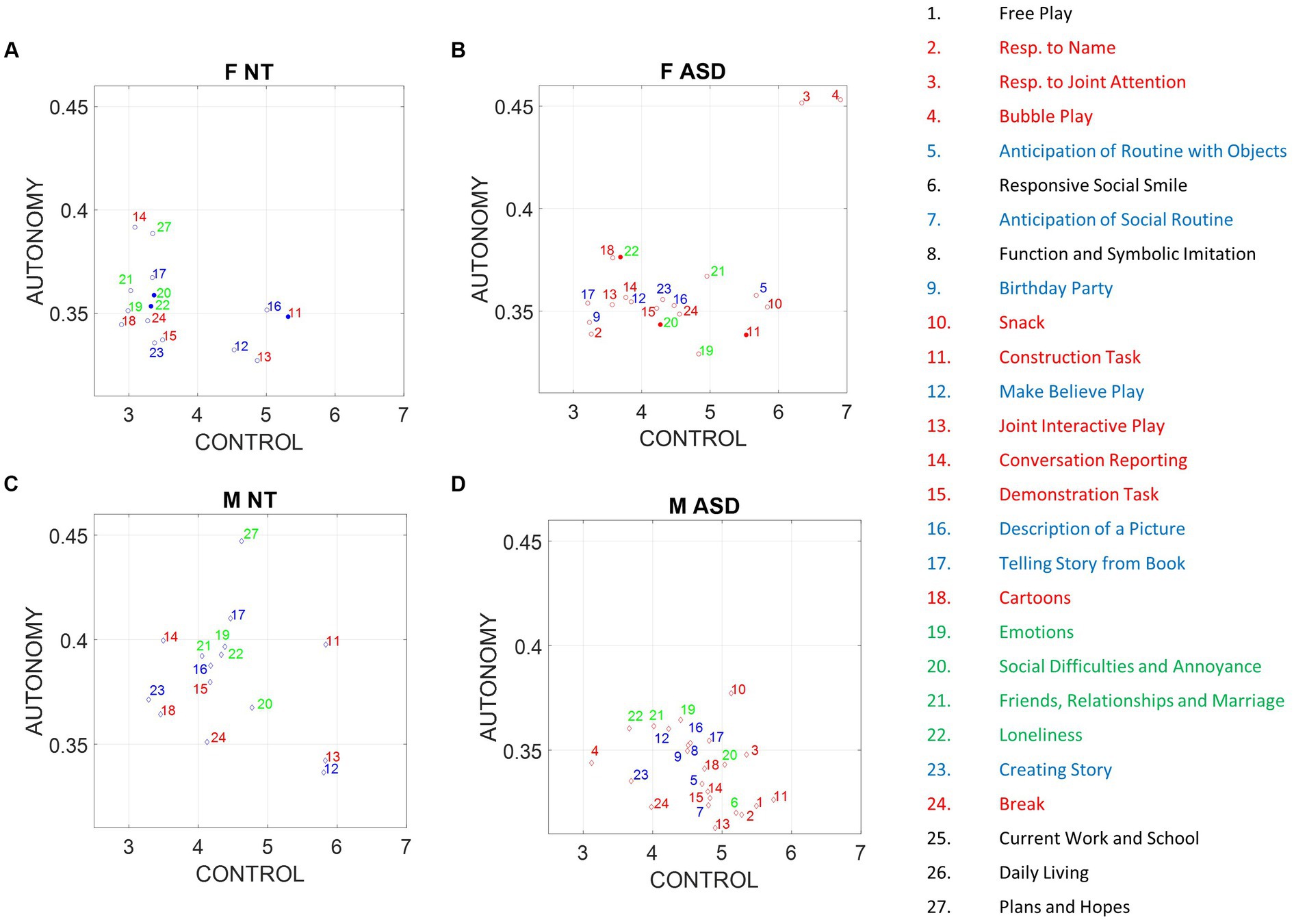
Figure 6. Differences between males and females in average index of autonomy versus control. Filled circles code non-significant differences at the 0.05 level, while non-filled circles denote significant differences between NT and ASD participants. (A) NT females. (B) ASD females. (C) NT males. (D) ASD males.

Notice that despite the non-significance in differences of emotional and socio-motor tasks, emotional tasks have broader spread in ASD females along the index of motor control than do NT females. In contrast, the index of motor autonomy has comparable spread for both. As socio-motor agency is defined as the ratio of index of motor autonomy/index of motor control, this implies that across these ADOS emotional tasks, NT females have more socio-motor agency than ASD females. In contrast to females, a statistically significant difference between the two male groups was observed for all tasks (according to non-parametric Kruskal–Wallis and t-test). ASD males shift significantly to lower values of the index of motor autonomy across all tasks, but visibly socio-motor tasks are deeply affected.

3.6 Motor controllability of an agent in a dyadic social interaction is inversely proportional to motor autonomy: leveraging socio-motor agency to protect the agent

In the Methods section, we defined what transfer entropy TY→Xkl between two processes X and Y is:

TY→Xkl=EtY→Xn+1,k,l    (6) tY→Xn+1,k,l=iynl;|xn+1|xnk

Equivalently, TE can be seen as the difference between the conditional entropy rate (which is equal to entropy rate for stationary processes) hX of process X and the generalized entropy rate hX,Y of X conditioning on the source Y (Prokopenko et al., 2013):

TY→Xkl=hX−hX,Y    (7)

With:

hX=−∑pxn+1xnklogpxn+1