Quantum imaging is the field of optics that aims to exploit the quantum properties of light to improve the performance of imaging systems [1]. Among the existing non-classical sources of light, those that emit pairs of entangled photons have proven to be particularly fruitful. For example, imaging with sensitivity below the shot-noise limit [2] and with resolution beating classical diffraction [3, 4] were both achieved with photon pair states. In addition, these sources also enabled the development of new imaging modalities. Examples include ghost imaging [5], imaging with undetected photons [6], entanglement-enabled holography [7] and Hong–Ou–Mandel microscopy [8].
Thanks to recent technological advancements, photon-pair-based imaging systems have become relatively simple to implement. Firstly, entangled photon pairs can be produced using spontaneous parametric down conversion (SPDC) [9]. This is a non-linear optical process that converts a single, high-energy photon, called the pump, into two lower energy photons, traditionally called the signal and idler. It does not require complex laser systems or highly specialised non-linear crystals. For example, many experiments—like the one presented here—use standard room-temperature 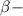 Barium Borate (BBO) crystals and a blue diode laser with a power of a few tens of milliwatts. Then, another factor that adds to the ease of implementation is the recent advent of commercial single-photon-sensitive cameras. Paired with advanced image processing techniques, these cameras enable the detection of photon coincidences across many transverse spatial positions simultaneously—a key measurement when working with entangled photon pairs. For example, electron-multiplying charge-coupled devices (EMCCDs) and single-photon avalanche diodes (SPADs) cameras have enabled the measurement of intensity correlations functions i.e. the
Barium Borate (BBO) crystals and a blue diode laser with a power of a few tens of milliwatts. Then, another factor that adds to the ease of implementation is the recent advent of commercial single-photon-sensitive cameras. Paired with advanced image processing techniques, these cameras enable the detection of photon coincidences across many transverse spatial positions simultaneously—a key measurement when working with entangled photon pairs. For example, electron-multiplying charge-coupled devices (EMCCDs) and single-photon avalanche diodes (SPADs) cameras have enabled the measurement of intensity correlations functions i.e. the 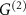 for photon pairs across thousands of spatial modes [10–13]. Despite this recent progress, the low brightness of SPDC sources (i.e. tens of picowatts) and long acquisition times (e.g. sometimes up to several h) still restrict their application to the laboratory environment and specific niche areas.
for photon pairs across thousands of spatial modes [10–13]. Despite this recent progress, the low brightness of SPDC sources (i.e. tens of picowatts) and long acquisition times (e.g. sometimes up to several h) still restrict their application to the laboratory environment and specific niche areas.
Besides generation and detection, it is also important to control how photon pairs and their characteristics (e.g. correlations) propagate within the optical system. For example, spatial light modulators (SLMs) can be placed in the pump beam or the photon pairs path to manipulate their spatial properties. They enable tailoring of the spatial entanglement between photons propagating in free space by manipulating their orbital angular spectrum [14–20], by shaping momentum-position correlations [21–23] or by tuning the pump spatial coherence [24, 25]. In addition, they can also be used to control photon pair propagation through complex media such as multimode fibers [26–28] and scattering layers [29–32]. These efforts are mainly directed towards applications in communication and information processing.
Though less explored, such control can also be of interest for quantum imaging. Indeed, if we consider the classical domain, light structuring has many applications in imaging, particularly in microscopy [33]. Examples range from structured illumination microscopy [34], which aims to improve imaging resolution by illuminating the sample with specifically tailored pattern of light, to adaptive optics (AO) [35] and wavefront shaping [36], which use an SLM to correct for optical aberrations and scattering, with applications also for contrast-enhanced and quantitative phase imaging [37, 38]. It is therefore interesting to see how we could apply these concepts to quantum imaging and it is within this context that this tutorial is written. Here, we describe two basic experiments that both use an SLM to shape the spatial correlations between entangled photon pairs. We detail their practical implementation and highlight their differences from their classical counterparts. Additionally, we show examples of applications that illustrate the practical uses of this two-photon shaping, including a case in imaging. We also include detailed descriptions of many of the key steps in this work in the supplementary material (S.M.), and provide example code in [39].
When shaping spatial correlations between pairs of photons, two experimental configurations can be considered. These are called the far-field (FF) and near-field (NF) shaping configurations, named for the position of the SLM relative to the nonlinear crystal. Note that in other works the configurations may instead be named for the position of the detector relative to the crystal. Figures 1(a), (b) shows schematics of these two configurations. In both cases a nonlinear crystal, a thin β-BBO crystal, is illuminated by a continuous-wave (CW) pump laser at 405 nm. This produces pairs of photons at 810 nm via Type I SPDC. Immediately after the crystal, the pump photons are filtered out using a 650 nm-cut-off long-pass filter, allowing only the photons of higher wavelengths generated by SPDC to propagate through the system. In practice, it is common to also include a short-pass filter in the pump beam's path before the crystal (not shown). This filter removes residual low-frequency light emitted by the laser, typically found at the output of blue diode lasers. After propagating through the system, the pairs are detected with an EMCCD camera, and the spatial correlations are measured following the method described in [12] (see also Methods). In both configurations, the pairs are controlled by a SLM that is positioned in a Fourier plane of the camera. A bandpass filter at 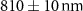 is used to remove ambient light and most non-degenerate pairs. Due to the phase matching conditions in the SPDC process, the photons at the plane of the crystal are strongly correlated in transverse position (r) and strongly anti-correlated in transverse momentum (k) [40]. Both of these types of correlations can be exploited, hence the two experimental configurations.
is used to remove ambient light and most non-degenerate pairs. Due to the phase matching conditions in the SPDC process, the photons at the plane of the crystal are strongly correlated in transverse position (r) and strongly anti-correlated in transverse momentum (k) [40]. Both of these types of correlations can be exploited, hence the two experimental configurations.
Figure 1. Experimental Setup. Spatially entangled photon pairs centred at 810 nm are produced via Type I spontaneous parametric down conversion (SPDC) using a collimated (0.8 mm diameter), continuous-wave laser at 405 nm and a thin 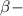 Barium Borate nonlinear crystal (NLC). Pump photons are filtered out by a long-pass filter (LP) at 650 nm. A bandpass filter at
Barium Borate nonlinear crystal (NLC). Pump photons are filtered out by a long-pass filter (LP) at 650 nm. A bandpass filter at 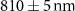 before the single-photon sensitive camera filters out any non-degenerate pairs. (a) Diagram of a far-field shaping configuration (FF) . The surface of the NLC is imaged onto the camera using two 4-f relays (
before the single-photon sensitive camera filters out any non-degenerate pairs. (a) Diagram of a far-field shaping configuration (FF) . The surface of the NLC is imaged onto the camera using two 4-f relays (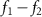 and
and  ). The spatial light modulator (SLM) is placed in the Fourier plane, or far-field, of the crystal. In this configuration, the photons are correlated at the camera, and anti-correlated at the SLM. (b) Diagram of a near-field shaping configuration (NF). The Fourier plane (FP) of the NLC, obtained via the lens f1, is imaged into the camera with two 4f relays (
). The spatial light modulator (SLM) is placed in the Fourier plane, or far-field, of the crystal. In this configuration, the photons are correlated at the camera, and anti-correlated at the SLM. (b) Diagram of a near-field shaping configuration (NF). The Fourier plane (FP) of the NLC, obtained via the lens f1, is imaged into the camera with two 4f relays (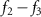 and
and  ). The SLM is placed in the conjugate plane to the surface of the NLC. In this configuration, the photons are anti-correlated at the camera, and correlated at the SLM. (c), (e) Direct intensity images from the camera in the FF shaping (c) and NF shaping (e) configurations. (d) Minus-coordinate projection of the measured
). The SLM is placed in the conjugate plane to the surface of the NLC. In this configuration, the photons are anti-correlated at the camera, and correlated at the SLM. (c), (e) Direct intensity images from the camera in the FF shaping (c) and NF shaping (e) configurations. (d) Minus-coordinate projection of the measured 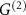 in the FF configuration. f, Sum-coordinate projection of the measured
in the FF configuration. f, Sum-coordinate projection of the measured 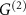 in the NF configuration. (c), (d) are from an acquisition of
in the NF configuration. (c), (d) are from an acquisition of 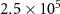 frames. (e), (f) are from an acquisition of
frames. (e), (f) are from an acquisition of 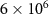 frames. M–mirror.
frames. M–mirror.
Download figure:
Standard image High-resolution imageThe FF shaping configuration is shown in figure 1(a). As suggested by the name, the SLM is positioned in a Fourier plane of the crystal, and its surface is imaged on the EMCCD. Since the pairs are correlated in position at the crystal plane, they are also correlated in position at the camera, so they arrive at approximately the same pixel. Figure 1(c) shows an example of the intensity at the camera. The shape of this is mostly dependent on the intensity profile of the pump. Figure 1(d) shows the so-called minus-coordinate projection of the measured 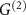 of the photon pairs. The way this is measured is detailed in the Methods section. It represents the probability of detecting both photons of a pair simultaneously on two pixels of the camera separated by a distance (
of the photon pairs. The way this is measured is detailed in the Methods section. It represents the probability of detecting both photons of a pair simultaneously on two pixels of the camera separated by a distance (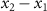 ,
, 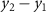 ), expressed in pixels. This type of measurement is essential when working with entangled photon pairs because it provides information about the spatial correlations between the pairs, something that an intensity image alone does not provide. A bright, narrow peak, as seen in the figure, indicates that the pairs are strongly correlated in position. This means that, when one photon in a pair is detected at a position
), expressed in pixels. This type of measurement is essential when working with entangled photon pairs because it provides information about the spatial correlations between the pairs, something that an intensity image alone does not provide. A bright, narrow peak, as seen in the figure, indicates that the pairs are strongly correlated in position. This means that, when one photon in a pair is detected at a position 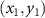 , there is a high probability to detect its twin within a very small area around this pixel
, there is a high probability to detect its twin within a very small area around this pixel 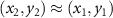 . For the measurement of the data shown in figure 1(d), the SLM did not display any phase mask.
. For the measurement of the data shown in figure 1(d), the SLM did not display any phase mask.
The NF shaping configuration is shown in figure 1(b). Here, the SLM is positioned in an conjugate plane to the surface of the crystal, and the Fourier plane of the crystal is imaged onto the camera. Now, due to momentum conservation in the SPDC process, the pairs arrive at the camera at diametrically opposite pixels, relative to the centre of the beam. Figure 1(e) shows an example of the intensity at the camera. Here, the typical SPDC ring can be seen. The thickness of this ring is proportional to the bandwidth of the pairs, and the radius is dependent on the angle between the pump optical axis and the normal to the crystal surface. Figure 1(f) shows the so-called sum-coordinate projection of the measured 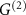 . The method of measuring this projection is also explained in the Methods section. It represents the probability of detecting two entangled photons at any pair of pixels of the camera
. The method of measuring this projection is also explained in the Methods section. It represents the probability of detecting two entangled photons at any pair of pixels of the camera 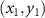 and
and 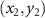 with a given barycenter value
with a given barycenter value 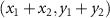 . For example, the central value of the sum-coordinate projection corresponds to the sum of all coincidence rates detected between pairs of pixels with a barycenter at (0, 0), which means all the pairs of pixels that are exactly anti-symmetric i.e.
. For example, the central value of the sum-coordinate projection corresponds to the sum of all coincidence rates detected between pairs of pixels with a barycenter at (0, 0), which means all the pairs of pixels that are exactly anti-symmetric i.e. 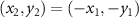 . In our experiment, the presence of an intense peak at the center indicates strong spatial anti-correlation. This means that when a photon from a pair is detected at a position
. In our experiment, the presence of an intense peak at the center indicates strong spatial anti-correlation. This means that when a photon from a pair is detected at a position 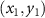 , there is a high probability that its twin will be detected within a very small area around the symmetric pixel
, there is a high probability that its twin will be detected within a very small area around the symmetric pixel 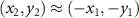 . No phase mask was displayed on the SLM to perform the measurement shown in figure 1(f). Finally, it is also important to remember that each configuration captures a different optical plane of the crystal on the camera. In FF-shaping configuration, it is the crystal surface, while in NF, it is the Fourier plane. This explains the slight difference in intensity shapes shown figures 1(c) and (e).
. No phase mask was displayed on the SLM to perform the measurement shown in figure 1(f). Finally, it is also important to remember that each configuration captures a different optical plane of the crystal on the camera. In FF-shaping configuration, it is the crystal surface, while in NF, it is the Fourier plane. This explains the slight difference in intensity shapes shown figures 1(c) and (e).
In this section we will describe the theory behind two-photon correlation shaping and compare it to classical shaping. When comparing classical and quantum imaging, it is useful to work with the spatial intensity correlation functions, specifically, the first and second order functions 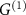 and
and 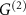 [41]. The quantities we measure in experiment can be written in terms of these, and they can also be used to derive expressions for propagation through an optical system.
[41]. The quantities we measure in experiment can be written in terms of these, and they can also be used to derive expressions for propagation through an optical system.
First we introduce the relevant measured quantities, and how they are written in terms of 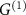 and
and 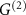 . When imaging and shaping with classical coherent light, the quantity we measure with the camera is the intensity of the electric field,
. When imaging and shaping with classical coherent light, the quantity we measure with the camera is the intensity of the electric field, 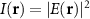 . This can be written in terms of the first-order spatial correlation function of the field as:
. This can be written in terms of the first-order spatial correlation function of the field as:


where 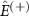 and
and 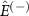 are the positive and negative frequency component of the quantum operator associated with the electric field, respectively. In practice, the intensity is conventionally measured using a camera by accumulating photons on each pixel.
are the positive and negative frequency component of the quantum operator associated with the electric field, respectively. In practice, the intensity is conventionally measured using a camera by accumulating photons on each pixel.
When working with photon pairs, the quantity that interests us the most is the second-order spatial correlation function of the intensity, 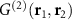 . This is also often referred as the joint probability distribution of the photons. It is written in terms of the second-order spatial correlation function of the field as:
. This is also often referred as the joint probability distribution of the photons. It is written in terms of the second-order spatial correlation function of the field as:
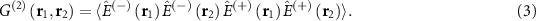
The 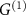 describes the field correlations and the second-order correlation function can be seen as the intensity-correlation analogue of this. In practice, it is measured by detecting photon coincidence between pairs of spatial positions
describes the field correlations and the second-order correlation function can be seen as the intensity-correlation analogue of this. In practice, it is measured by detecting photon coincidence between pairs of spatial positions 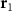 and
and 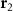 . As detailed in [12], it can also be reconstructed by measuring the intensity covariance between pairs of pixels on a camera. Assuming we have pure two-photon states,
. As detailed in [12], it can also be reconstructed by measuring the intensity covariance between pairs of pixels on a camera. Assuming we have pure two-photon states, 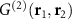 gives the probability of simultaneously detecting two photons from a pair at positions
gives the probability of simultaneously detecting two photons from a pair at positions 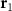 and
and 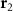 . This quantity is related to the spatial two-photon wave function φ, as:
. This quantity is related to the spatial two-photon wave function φ, as: 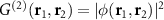 . In this way, φ is to
. In this way, φ is to 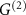 in the two-photon case as the field E is to I in the case of coherent light.
in the two-photon case as the field E is to I in the case of coherent light.
Since we want to see how we can shape the distributions I and 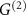 , it is useful to know how they propagate. Any linear system can be described by its coherent point-spread function (PSF). Given the PSF, written
, it is useful to know how they propagate. Any linear system can be described by its coherent point-spread function (PSF). Given the PSF, written 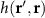 , the correlation functions can be propagated through any linear system as:
, the correlation functions can be propagated through any linear system as:
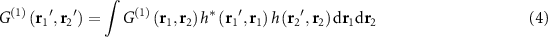
and
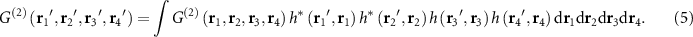
For example, let us consider the two experimental configurations described in figures 1(a) and (b) (NF and FF shaping). In both cases, the camera is positioned in a Fourier plane of the SLM. Since the action of the SLM is to impart a phase profile, 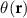 , onto the beam, the PSF associated with the propagation between the SLM (shaping plane) and the camera (detection plane) can be written as
, onto the beam, the PSF associated with the propagation between the SLM (shaping plane) and the camera (detection plane) can be written as
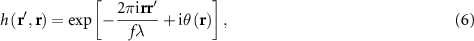
where f is the focal length of the lens immediately after the SLM, λ is the wavelength of the light being used, r and 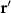 are the transverse coordinates in the SLM and camera plane, respectively. Note that in each configuration there are in reality several lenses performing a magnification in addition to the Fourier transform, which can be taken into account by changing the effective value of f in the previous equation.
are the transverse coordinates in the SLM and camera plane, respectively. Note that in each configuration there are in reality several lenses performing a magnification in addition to the Fourier transform, which can be taken into account by changing the effective value of f in the previous equation.
For a perfectly spatially coherent source, e.g. a laser, the first-order correlation function is given by

where 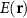 is the electric field at position r in the SLM plane and
is the electric field at position r in the SLM plane and  denotes the complex conjugate. Thus, from equations (2) and (4), the intensity after propagation through the system is given by
denotes the complex conjugate. Thus, from equations (2) and (4), the intensity after propagation through the system is given by

Using equation (6) and simplifying, we obtain the intensity at the camera
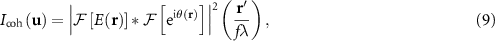
where ![$\mathcal[\ldots]$](https://content.cld.iop.org/journals/2515-7647/6/3/033001/revision2/jpphotonad50b1ieqn48.gif) is the 2-dimensional Fourier transform, and
is the 2-dimensional Fourier transform, and  denotes the 2-dimensional convolution operation. This is the expected result from Fourier-optics and says that the field measured at the camera is simply the (scaled) Fourier transform of the phase mask on the SLM. The intensity correlation function for a coherent source is simply
denotes the 2-dimensional convolution operation. This is the expected result from Fourier-optics and says that the field measured at the camera is simply the (scaled) Fourier transform of the phase mask on the SLM. The intensity correlation function for a coherent source is simply

For coherent light, 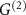 can thus be fully computed from the intensity measurements and therefore does not contain any additional information.
can thus be fully computed from the intensity measurements and therefore does not contain any additional information.
Now let us consider a two-photon state. In the input plane i.e. the SLM plane, we can write our state in the position basis as

where 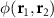 is the two-photon wavefunction expressed in the position basis,
is the two-photon wavefunction expressed in the position basis,  and
and 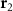 are the transverse positions in the SLM plane for photon 1 and photon 2 respectively. In our case, both photon have the same polarisation and spectral frequency. This results from the pair generation process that we have chosen to use in our experiments, which is Type I SPDC. In this simplified notation,
are the transverse positions in the SLM plane for photon 1 and photon 2 respectively. In our case, both photon have the same polarisation and spectral frequency. This results from the pair generation process that we have chosen to use in our experiments, which is Type I SPDC. In this simplified notation, 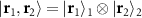 denotes the state in which photon 1 is at position
denotes the state in which photon 1 is at position  and photon 2 is at position
and photon 2 is at position 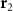 . The
. The 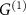 for such a state is given by
for such a state is given by
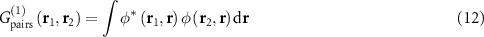
and the direct intensity is then

Additionally, one can compute the second-order field correlations as:
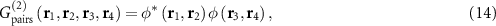
giving the intensity correlations:

Using equations (4) and (5), 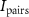 and
and 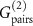 can be expressed in the camera plane:
can be expressed in the camera plane:
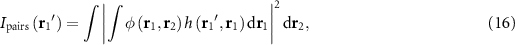
and
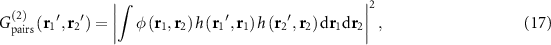
where 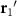 and
and 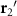 are the transverse positions in the camera plane,
are the transverse positions in the camera plane, 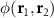 is the two-photon wavefunction in the SLM plane and h is the PSF.
is the two-photon wavefunction in the SLM plane and h is the PSF.
Now we consider the two shaping configurations shown in figures 1(a) and (b). In both cases, the imaging system from the SLM to the camera, h, is the same (up to a different magnification factor), but the input states are different. We start with the configuration of figure 1(a). Here, the SLM is positioned in the Fourier plane of the crystal. In our experimental conditions the collimated pump beam diameter is much larger than the crystal thickness. Therefore, we can assume that photon pairs are near-perfectly anti-correlated in the plane immediately before the SLM [42]. That is, the wavefunction 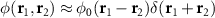 , where φ0 is the amplitude envelope of the two-photon wavefunction in the crystal Fourier plane. It is linked to the intensity measured in the SLM plane as
, where φ0 is the amplitude envelope of the two-photon wavefunction in the crystal Fourier plane. It is linked to the intensity measured in the SLM plane as 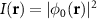 . In practice, it takes the shape of a disk or a ring, as shown in figure 1(e), and its spatial phase is assumed to be uniform. After performing the change of variables
. In practice, it takes the shape of a disk or a ring, as shown in figure 1(e), and its spatial phase is assumed to be uniform. After performing the change of variables 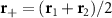 and
and 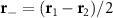 , the intensity correlation in the camera plane can be expressed as
, the intensity correlation in the camera plane can be expressed as
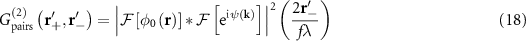
where  and all global constants are omitted for clarity.
and all global constants are omitted for clarity.
Now we consider the configuration in figure 1(b). Here we are imaging the crystal plane onto the SLM. Since we have thin crystal and a large pump diameter, we can assume that the photon pairs are perfectly correlated, i.e. 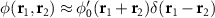 [42], where
[42], where 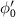 is the amplitude envelope of the two-photon wavefunction in the crystal plane. It is linked to the intensity measured in the SLM plane as:
is the amplitude envelope of the two-photon wavefunction in the crystal plane. It is linked to the intensity measured in the SLM plane as: 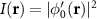 . In practice, it takes the shape of the pump beam, as shown in figure 1(c), and its spatial phase is assumed to be uniform. Then, the intensity correlations at the camera are given by
. In practice, it takes the shape of the pump beam, as shown in figure 1(c), and its spatial phase is assumed to be uniform. Then, the intensity correlations at the camera are given by

By performing a similar propagation calculation with 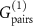 , we find that the intensities on the camera plane are spatially uniform in both configurations and do not depend on the phase displayed on the SLM i.e.
, we find that the intensities on the camera plane are spatially uniform in both configurations and do not depend on the phase displayed on the SLM i.e. 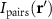 is a constant. Thus, as would be the case for a perfectly spatially incoherent source, the SLM does not modulate the intensity measured in the Fourier plane. This near-perfect spatial incoherence is indeed observed experimentally and is a consequence of our experimental conditions, specifically the use of a collimated pump with a diameter much larger than the thickness of the crystal. By changing the crystal and the illumination conditions [43], it would be possible to work in an intermediate regime with partially spatially coherent light, allowing modulation of both the intensity and the intensity correlations.
is a constant. Thus, as would be the case for a perfectly spatially incoherent source, the SLM does not modulate the intensity measured in the Fourier plane. This near-perfect spatial incoherence is indeed observed experimentally and is a consequence of our experimental conditions, specifically the use of a collimated pump with a diameter much larger than the thickness of the crystal. By changing the crystal and the illumination conditions [43], it would be possible to work in an intermediate regime with partially spatially coherent light, allowing modulation of both the intensity and the intensity correlations.
Comparing equations (9), (18) and (19) we see that the spatial intensity correlations can be shaped in a manner that is almost equivalent to spatial intensity shaping in the classical case. Under our experimental conditions, ![$\mathcal[E(\mathbf)]$](https://content.cld.iop.org/journals/2515-7647/6/3/033001/revision2/jpphotonad50b1ieqn73.gif) ,
, ![$\mathcal[\phi_0(\mathbf)]$](https://content.cld.iop.org/journals/2515-7647/6/3/033001/revision2/jpphotonad50b1ieqn74.gif) and
and ![$\mathcal[\phi_0^}(\mathbf)]$](https://content.cld.iop.org/journals/2515-7647/6/3/033001/revision2/jpphotonad50b1ieqn75.gif) are very sharply peaked, and can therefore be approximated as Dirac Delta functions. This allows for the simplification of the equations to focus on the role played by the SLM:
are very sharply peaked, and can therefore be approximated as Dirac Delta functions. This allows for the simplification of the equations to focus on the role played by the SLM:
![$I_\textrm = \left|\mathcal\left[\textrm^\theta(\mathbf)}\right]\right|^2$](https://content.cld.iop.org/journals/2515-7647/6/3/033001/revision2/jpphotonad50b1ieqn76.gif) ,Pairs—FF shaping:
,Pairs—FF shaping:
Comments (0)